Very nice instructions, I agree with just about everything, However the best advice I can give after experimenting with open baffles, is forget about it. It can give good results, but way too much trial and error and way too much room dependent.
-
Welcome to ASR. There are many reviews of audio hardware and expert members to help answer your questions. Click here to have your audio equipment measured for free!
Open baffle speaker pitfalls
- Thread starter ctrl
- Start date
For a two months I'm using these. 38 x 50 cm baffle, Eminence Beta-12CX:n and Eminence ASD1001 elements.
One great speaker guy in Finland discovered that these Eminences give good response 30 degrees off center.
Kit is sold by Uraltone store and it's 275 EUR with filter parts.
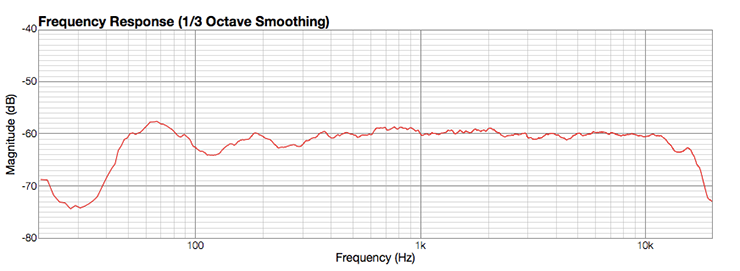
He measured response like this. I don't measure.
I use one subwoofer. Making right stands now.
One great speaker guy in Finland discovered that these Eminences give good response 30 degrees off center.
Kit is sold by Uraltone store and it's 275 EUR with filter parts.
He measured response like this. I don't measure.
I use one subwoofer. Making right stands now.
- Thread Starter
- #23
Glad you're happy with your OB speaker, but could it be that you accidentally posted in the wrong thread?
General experiences with OB speakers are collected in the thread "Open Baffle speakers".
This thread is about what problems can occur, especially when deviating from the classic OB design (flat baffle).
That some manufacturers call their OB speakers the "pinnacle of open baffle loudspeaker designs", but the radiation is anything but that of a dipole (yet what buyers usually expect) and in some cases more like a speaker design nightmare.
Hmm, I thought the thread is right. No nightmares, sounds great. I can't think out any nightmares with it.
And it deviates from classic design for sure. And radiation is diapole for sure.
Of cource, it depends on someones's thoughts about sound.
And it deviates from classic design for sure. And radiation is diapole for sure.
Of cource, it depends on someones's thoughts about sound.
- Thread Starter
- #25
The baffle itself is classic (though small in dimensions). Unusual is the vertical inclination of the baffle, which provides for a flatter response of the direct sound on the reference axis of the speaker - instead of keeping the often very wavy on-axis frequency response of a coaxial loudspeaker as a reference axis.
For this, the vertically also pronounced dipole behavior of the woofer is also tilted by 30 °, which leads to more floor and ceiling reflections as a compromise.
Yep, that's right. Of course, only up to the crossover frequency of the compression driver, from there it's a normal monopole speaker.
Last edited:
So if I added a baffle step compensation crossover network, it goes to the woofer and it lowers the output of the woofer for the whole section on a frequency response graph where the woofer is emitting a dipole radiation pattern? Or a carotid? Carotid is a beamed / laser radiation pattern?
At the end of this article http://musicanddesign.speakerdesign.net/u_frame.html is the following, which indicates the damped uframe (if you are careful) is effectively as good as an hframe that is twice the size … it seems to me that a uframe like this crossed out just above 100 hz could hand off to another identical driver in a sealed box and provide extended low frequency response beyond 500 hz (assuming 15” drivers) with a good match on dispersion?
Last edited:
@ctrl, what is the impact on response if the shape of the H-frame is modified, but….. all sides remain parallel (no V-frame type mods) and total volume of the H-frame chambers (front and rear) remain consistent with standard un-modified design. See the side-view examples attached.
Attachments
- Thread Starter
- #29
That's an interesting question. My guess would be that for cases two and three on your sketch, the first in-phase on-axis resonance is less pronounced and the frequency range is a tiny bit broader.
At the moment I don't have time to design simulations to match your sketch, but have put it on my to-do list.
- Thread Starter
- #31
8. H frame with angled side walls
These are the simulations to @ajar's question in post#28.
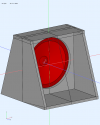
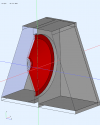
For this purpose, a "normal" H-frame with a 15'' woofer (TSP from BIANCO-15OB350 used) with the dimensions WxHxD 44x44x40 cm in 2m distance was simulated first.
The woofer is mounted from behind on the separating wall.
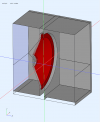
Secondly, an H-frame with angled side wall was simulated. Only the depth was changed to 20 cm at the top and 50 cm at the bottom.
For the evaluation, the order is always first the image of the normal H-frame, then the H-frame with angled side walls. Ignore all FR over 2kHz because of the used simulation limit.
First, we compare the on-axis frequency responses of the front (blue), rear (green) and sum (red) of the radiated sound.
As already suspected, the resonance (caused by the walls in front of and behind the woofer) is a little better under control in the version with "angled side walls", but since the resonance is outside the range of use, this is not a real advantage.
What you can also see is that the sensitivity in the low bass range is slightly better with the normal H-frame version. This becomes especially obvious when comparing the on-axis frequency responses at the same power level:
At 100Hz the SPL of the normal H-frame is about 1.2 dB higher than the "angled version".
The desired dipole radiation is present in both versions up to the usage limit of about up to 150Hz. Here the sonograms of the on-axis normalized FR:
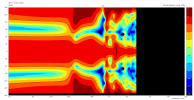
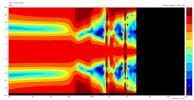
As usual, dipole radiation breaks down completely near resonance and should be avoided at all costs.
The use of "angled side walls" brings no real advantage, but slightly less SPL in the low frequency range and the speaker with the angled side walls requires a much larger area of floor space than the normal H-frame.
These are the simulations to @ajar's question in post#28.
For this purpose, a "normal" H-frame with a 15'' woofer (TSP from BIANCO-15OB350 used) with the dimensions WxHxD 44x44x40 cm in 2m distance was simulated first.
The woofer is mounted from behind on the separating wall.
Secondly, an H-frame with angled side wall was simulated. Only the depth was changed to 20 cm at the top and 50 cm at the bottom.
For the evaluation, the order is always first the image of the normal H-frame, then the H-frame with angled side walls. Ignore all FR over 2kHz because of the used simulation limit.
First, we compare the on-axis frequency responses of the front (blue), rear (green) and sum (red) of the radiated sound.
As already suspected, the resonance (caused by the walls in front of and behind the woofer) is a little better under control in the version with "angled side walls", but since the resonance is outside the range of use, this is not a real advantage.
What you can also see is that the sensitivity in the low bass range is slightly better with the normal H-frame version. This becomes especially obvious when comparing the on-axis frequency responses at the same power level:
At 100Hz the SPL of the normal H-frame is about 1.2 dB higher than the "angled version".
The desired dipole radiation is present in both versions up to the usage limit of about up to 150Hz. Here the sonograms of the on-axis normalized FR:
As usual, dipole radiation breaks down completely near resonance and should be avoided at all costs.
The use of "angled side walls" brings no real advantage, but slightly less SPL in the low frequency range and the speaker with the angled side walls requires a much larger area of floor space than the normal H-frame.
- Thread Starter
- #32
9. U-frame resonance side effects
How difficult it is to "avoid" the U-frame resonance should have been mentioned in the opening post, as it was one of the reasons to start this thread. This means that even drivers that are not directly in the U-frame but, for example, a little above it, of course still excite the resonance.
For this purpose we will look at the simulation of a loudspeaker with an angled U-frame.
In the lower part of the baffle there are normally two 15'' woofers, which were omitted for the simulation. As we know, the crossover frequency for these woofers in the U-frame has to be chosen very low - mostly at 60-100Hz (below the occurring cabinet resonance / short transmission line resonance).
One could be under the misapprehension that the upper mid-bass driver (or coaxial driver), which is used from about 80Hz upwards, will not have any problems with the angled U-frame, since there are hardly any side walls at its height.
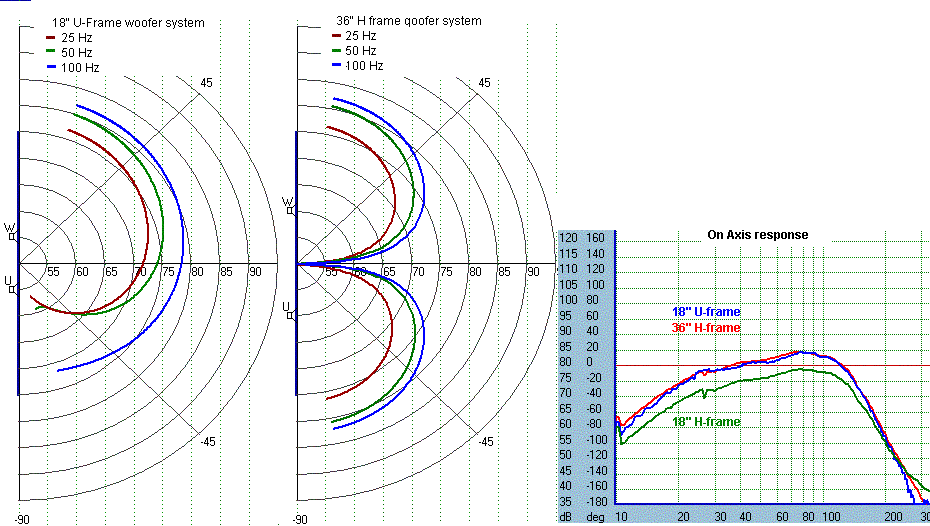
We compare the radiation of the "angled U-frame" with the classic OB concept. Again, the two woofers are omitted for the simulation:
First, we compare the on-axis frequency responses (red summed FR, blue woofer front FR, green woofer rear FR). First is always the classic OB, second the "angled U-frame":
The classic OB speaker shows an almost perfect frequency response with the usual fast SPL drop towards low frequencies.
With the angled U-frame, on the other hand, the fundamental resonance of a "short" transmission line (the side walls form a kind of TL) is excited by the woofer. This in turn causes massive resonances in the rear emitted sound. Which in the sum with the sound radiated to the front causes a wavy frequency response (red curve).
This becomes particularly clear when comparing the on-axis FR of both concepts:
Unfortunately, this is not the real problem. The problem is the collapse of the dipole radiation in the area around the fundamental resonance and the uneven radiation caused by the harmonics.
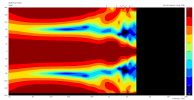
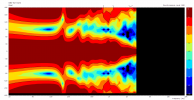
For those who can do little with the normalized sonograms, here are simply the horizontal frequency responses of the two concepts from 0° to 180° in 20° steps.
I think you can see nicely how the U-frame concept causes an abrupt change in radiation in the range 130-230Hz and that this disturbance in the radiation of the speaker continues in attenuated form around the harmonics. Whereas the classic OB speaker continues to show perfect dipole radiation.
How difficult it is to "avoid" the U-frame resonance should have been mentioned in the opening post, as it was one of the reasons to start this thread. This means that even drivers that are not directly in the U-frame but, for example, a little above it, of course still excite the resonance.
For this purpose we will look at the simulation of a loudspeaker with an angled U-frame.
In the lower part of the baffle there are normally two 15'' woofers, which were omitted for the simulation. As we know, the crossover frequency for these woofers in the U-frame has to be chosen very low - mostly at 60-100Hz (below the occurring cabinet resonance / short transmission line resonance).
One could be under the misapprehension that the upper mid-bass driver (or coaxial driver), which is used from about 80Hz upwards, will not have any problems with the angled U-frame, since there are hardly any side walls at its height.
We compare the radiation of the "angled U-frame" with the classic OB concept. Again, the two woofers are omitted for the simulation:
First, we compare the on-axis frequency responses (red summed FR, blue woofer front FR, green woofer rear FR). First is always the classic OB, second the "angled U-frame":
The classic OB speaker shows an almost perfect frequency response with the usual fast SPL drop towards low frequencies.
With the angled U-frame, on the other hand, the fundamental resonance of a "short" transmission line (the side walls form a kind of TL) is excited by the woofer. This in turn causes massive resonances in the rear emitted sound. Which in the sum with the sound radiated to the front causes a wavy frequency response (red curve).
This becomes particularly clear when comparing the on-axis FR of both concepts:
Unfortunately, this is not the real problem. The problem is the collapse of the dipole radiation in the area around the fundamental resonance and the uneven radiation caused by the harmonics.
For those who can do little with the normalized sonograms, here are simply the horizontal frequency responses of the two concepts from 0° to 180° in 20° steps.
I think you can see nicely how the U-frame concept causes an abrupt change in radiation in the range 130-230Hz and that this disturbance in the radiation of the speaker continues in attenuated form around the harmonics. Whereas the classic OB speaker continues to show perfect dipole radiation.
Last edited:
- Thread Starter
- #33
9b. Addition
One more small addition to point 9. "U-frame resonance side effects".
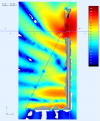
We can look at the sound pressure level distribution of the loudspeaker and see in detail what happens when the bass-midrange driver excites the cabinet resonance.
1) The first image is a sketch of the lateral cut of the speaker.
2) Then the SPL distribution at 90Hz where the speaker shows dipole behavior.
3) The SPL distribution at 150Hz shows the fundamental resonance of the speaker cabinet. The simulations take place in the free field (4pi room). Possibly, one should make an additional simulation with an boundary surface (here floor) to get the effects of the boundary reinforcement of resonances on the FR.
4) First harmonic about 300Hz
5) Resonances at 425Hz
6) Resonances at 1kHz
For comparison we have a look at the classic OB loudspeaker at the same frequencies and view:
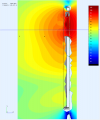
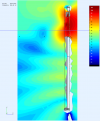
Above 200Hz there is increasing interference with the baffle, but this is classes lower than with the angled U-frame.
So, if you are looking for perfection, then U-frame speakers are recommended only under very specific conditions.
One more small addition to point 9. "U-frame resonance side effects".
We can look at the sound pressure level distribution of the loudspeaker and see in detail what happens when the bass-midrange driver excites the cabinet resonance.
1) The first image is a sketch of the lateral cut of the speaker.
2) Then the SPL distribution at 90Hz where the speaker shows dipole behavior.
3) The SPL distribution at 150Hz shows the fundamental resonance of the speaker cabinet. The simulations take place in the free field (4pi room). Possibly, one should make an additional simulation with an boundary surface (here floor) to get the effects of the boundary reinforcement of resonances on the FR.
4) First harmonic about 300Hz
5) Resonances at 425Hz
6) Resonances at 1kHz
For comparison we have a look at the classic OB loudspeaker at the same frequencies and view:
Above 200Hz there is increasing interference with the baffle, but this is classes lower than with the angled U-frame.
So, if you are looking for perfection, then U-frame speakers are recommended only under very specific conditions.
Hello
@ctrl thank you, your simulations are interesting especially the information if a design provides some monopol behavior or not. This information is really valuable. One big question remains unanswered: the performance below Schroeder Frequency of such a construction in a real room with different placements. From my understanding the simulation didn't contain the floor or any other potential wall next to the speaker. My interpretation of these simulation is that they are only useful if you can assume how a room/ placement will alter the frequency response. If you can't do that than these simulations can't help you much to choose a good design for your purpose.
We all know a bit about optimal placement of monopol subwoofers in rooms but it is very hard to think of a clever placement of a specific dipole bass in a room. You have to be aware that you create a more complex wave field in the room and that you can induce the room modes by different placements compared with a monopol. But I honestly don't know how to place one or even two dipole subwoofers cleverly in a room. I did it with trial and error with my dipol subwoofer.
If you ever heard any dipole subwoofer you will observe that the sound is very different compared with a similar in room equalized closed box or bass reflex box. I learned that the difference of the flow resistance can be hear / felt in bass frequencies due to the difference of air flow. I am wondering if this is the main cause why dipol subwoofers sound more soft and should be given a few dB more bass boast compared to a closed box.
@ctrl thank you, your simulations are interesting especially the information if a design provides some monopol behavior or not. This information is really valuable. One big question remains unanswered: the performance below Schroeder Frequency of such a construction in a real room with different placements. From my understanding the simulation didn't contain the floor or any other potential wall next to the speaker. My interpretation of these simulation is that they are only useful if you can assume how a room/ placement will alter the frequency response. If you can't do that than these simulations can't help you much to choose a good design for your purpose.
We all know a bit about optimal placement of monopol subwoofers in rooms but it is very hard to think of a clever placement of a specific dipole bass in a room. You have to be aware that you create a more complex wave field in the room and that you can induce the room modes by different placements compared with a monopol. But I honestly don't know how to place one or even two dipole subwoofers cleverly in a room. I did it with trial and error with my dipol subwoofer.
If you ever heard any dipole subwoofer you will observe that the sound is very different compared with a similar in room equalized closed box or bass reflex box. I learned that the difference of the flow resistance can be hear / felt in bass frequencies due to the difference of air flow. I am wondering if this is the main cause why dipol subwoofers sound more soft and should be given a few dB more bass boast compared to a closed box.
Last edited:
- Thread Starter
- #35
The simulations can show when a design is flawed. This is something you want to avoid at all costs, regardless of the room in which the loudspeaker is operated.
When designing speakers with U- or V-frame design, one must be extremely careful that the crossover frequency of the driver whose low-frequency output is to be increased is well below the cabinet resonance and that other drivers cannot "indirectly" excite this resonance.
As shown in post#20, in the worst case, the directivity of the speaker changes very quickly, very extremely in a certain frequency range, if U- and V-frame designs are used carelessly.
How much this will be audible is likely to depend on the room and individual tolerances to fluctuating sound power.
I agree, these simulations can't help you with this problem either.
But that is not the goal of the thread. Rather, it should make potential buyers or DIY builders aware of possible problems with certain OB speaker designs.
I agree with most of your statements. I also really like the insights of your simulations so please don't stop doing such things. But from my point of view the simulations and measurement of the bass frequency response below and slightly above the Schroeder Frequency without taking the room into account is almost pointless for praxis (at least if we don't have knowledge about typical behavior of different bass radiation pattern in our listening room).
If you add a solid floor to all of your simulations the frequencies which show "omnidirectional" behavior will change. Adding a back wall not to far away will again change where you have "omnidirectional" behavior...
My devils advocate question is therefore what is the point for praxis of simulating the bass frequency response of a dipole speaker without taking the room into account? If you buy/ built a design which looks good in free space it might not be the design which will measure well in your room.
In the mid to high frequencies it is for shure useful since we can distinguish the first wavefront from reflections to some degree.
If you add a solid floor to all of your simulations the frequencies which show "omnidirectional" behavior will change. Adding a back wall not to far away will again change where you have "omnidirectional" behavior...
My devils advocate question is therefore what is the point for praxis of simulating the bass frequency response of a dipole speaker without taking the room into account? If you buy/ built a design which looks good in free space it might not be the design which will measure well in your room.
In the mid to high frequencies it is for shure useful since we can distinguish the first wavefront from reflections to some degree.
Philbo King
Addicted to Fun and Learning
- Joined
- May 30, 2022
- Messages
- 792
- Likes
- 1,114
@ctrl Thanks a bunch for doing these simulations. This is very helpful!
- Joined
- Jun 5, 2016
- Messages
- 3,129
- Likes
- 5,282
Thanks for running all those sims and explaining them in a clear manner.
I trust the sims in free space, but - in small rooms is 130-230Hz really a region where radiation pattern is relevant?
Put another way, does the change in directivity materially impact how the system excites room modes, and thus the steady state response, in a small room? I recall John Kreskovsky (apologies if I butchered that) eventually came around to more-or-less endorse Earl Geddes' views on the topic. OTOH, his final published design was also a fully OB speaker, so...
Last edited:
- Thread Starter
- #40
It is simply not possible to create a simulation or measurement for every listening room in the world.
Therefore, it is standard that loudspeakers are measured under free field conditions and so that simulations can be compared with real measurements, these are also simulated under free field conditions - why should dipole speaker be the exception? (it's rhetorical don't answer
)The CTA-2034-A standard explicitly states under 5.1: "Measurements should be made in a reflection-free environment".
Further it says "Amplitude response measurements shall be made at 10° intervals on one horizontal and one vertical orbit around the DUT." Which is also fulfilled by the simulations. The virtual mic measures the simulation in 2m distance as stated in CTA-20234-A. The only thing I don't do is to convert the sound pressure level back to 1m.
It would certainly be interesting to simulate how different speaker concepts excite a sample room if the speaker position in the room is always identical, but that's not the goal of the thread.
If free field measurements or simulations in the bass-midrange show you errors in the radiation and directivity, then the probability that this speaker will cause problems in your listening room (and measure less well) is much higher than a speaker without design flaws.
Since in this frequency range in most listening rooms mainly the standing waves of the room modes dominate, tiny details in the radiation pattern are certainly not decisive.
But here we are talking about dipole radiation, which in the best case less excites the laterally room modes (and to a certain degree vertical) - in theory. If one would agree with your statement, then a dipole concept below the Schroeder frequency would not make sense.
What the simulations show is that with poorly designed U- and V-frame concepts, the dipole radiation is broken up in the region of the fundamental resonance (and the harmonics in some cases) of the open cabinet, and becomes omnidirectional for a certain frequency range - i.e., excites the lateral room modes more strongly.
Here again the simulation of example 1 from the OP, which is based on a real loudspeaker (woofer XO is likely 800-1200 Hz). The frequency responses normalized to the on-axis FR show that the lateral 90° radiation is even increased by up to 3dB in the range 170-300Hz compared to the on-axis FR.
In post#20 it was shown for another example how much the effects also show up in the CTA-2034 graph.
For U- or V-frame OB speakers, if the crossover frequency is not below the cabinet fundamental resonance, there are massive, likely audible problems - whether they bother the individual listener or whether this is simply "seen" as another "room resonance" is likely to vary.
With indirect excitation by a driver, for example, sitting above an angled U-frame, as shown in post#32, the effect is of course not so severe - but far from insignificant compared to the classic OB concept:
We can additionally have a look at the comparison of classic OB speaker vs angled U-frame OB speaker (see post#32) as CTA-2034 graph (ignore all stuff >2kHz, simulation limit):
How serious and how important the affected frequency range is, everyone must decide for themselves.
If the resulting fundamental resonance is ignored in U- and V-frame speaker designs, it's a serious design flaw, hence the word "pitfall" (that may not be correct wording - English is not my native language). "trade-off" does not do justice to the severity of the error - IMO.
Last edited:
Similar threads
