What is group delay?

[Holmz]

No. Simply no.

Group delay is time delay. Group delay is often described as the delay of the amplitude envelope ... which is a fancy word for time delay.

Does this help?

Technical Support: N5242B PNA-X Microwave Network Analyzer

The PNA-X vector network analyzer offers the world's most integrated and flexible microwave test platform for active device characterization.

na.support.keysight.com

Whether or not it is a time delay, it is usually expressed mathematically as the derivative of phase with respect to frequency.

Then they further confound easy of understanding with the term minimum phase, which appears to be something like…
“lets remove the bulk of the delay so that the phase is not wrapped around the axel.”
- And what is left is easier to see as a wriggle around a mostly flattened phase.

 

[witwald]

also worth keeping in mind that in natural objects (including speakers/headphones/cartridges) - most (but not all) frequency/phase relationships are minimum phase...

It should be kept in mind that it's the individual loudspeaker drivers that are minimum phase (e.g., woofers, midranges, tweeters). Once a crossover network is added, the combined multi-way system is generally no longer minimum phase. Approximate exceptions do exist, such as two-way loudspeaker systems utilising first-order crossover networks.

In digital EQ however, we can apply "linear phase" filters - which adjust ONLY the frequency/amplitude and leave the time domain (phase) alone.

Linear-phase filters add a constant time delay, which ensures that the waveform shape is maintained, subject of course to any filtering reduction of the frequency content.

 

[Holmz]

It should be kept in mind that it's the individual loudspeaker drivers that are minimum phase (e.g., woofers, midranges, tweeters). Once a crossover network is added, the combined multi-way system is generally no longer minimum phase. Approximate exceptions do exist, such as two-way loudspeaker systems utilising first-order crossover networks.

…

Can you expound on that some more please?

(The term and the descriptions usually have left me confused in the past.)

 

[dc655321]

Can you expound on that some more please?

(The term and the descriptions usually have left me confused in the past.)

Minimum phase components arranged in series produce a minimum phase system.

Those same components arranged in parallel (as in an N-way loudspeaker), may no longer constitute a minimum phase system.

 

[Frgirard]

Gearspace.com - View Single Post - Linear phase pre-ringing: Does it effect all frequencies?

Post 15367574 -Forum for professional and amateur recording engineers to share techniques and advice.

gearspace.com

 

[solderdude]

Minimum Phase

Audibility of group delay at low frequencies

Audibility of group delay at low frequencies: https://www.kvalsvoll.com/…/audibility-of-group-delay-at-l…/ It is then confirmed and proven that group delay at low frequencies is audible. This means timing - GD, phase - matter for sound in the bass range, it is not sufficient to tune towards a...

www.audiosciencereview.com

Group delay explanation and measurement (in audio)

Group delay is often confused with phase delay, and for good reasons. Here is a clear group delay explanation, and measurement using ARTA.

audiojudgement.com

 

[DSJR]

In a box speaker, I always equated it with Booooooooooom!

Maybe that's totally wrong, but to me that's an easily audible artefact often exhibited in UK-porty domestic speakers...

 

[dasdoing]

This means that if you use a minimum phase filter (standard "analogue" filter behaviour) to correct frequency response non linearities, you will also correct phase response non linearities. Your end result will be fully corrected in time, amplitude, frequency.

this might be mosly true in an anechoic chamber......but in a room you have a big excess phase component. all DRC software only corrects phase of the excess phase component. the term "mixed phase" filter is actualy used

 

[FrantzM]

Hi

With apologies to the many people who have taken their times to explain it, the answers so far have been of the circular nature , furthering the confusion. Once we start delving into "minimum Phase", "linear phase" and the likes, we are deep in System theory and have not explained to the layman what "Group Delay" is and how relevant it is in audio, where the jury seems to be still out of its audibility...
All this, makes me feel that I need to go back to my System and Signal Theory textbooks, and try to form an understanding. By the time I would make some sense of the concept, I am sure the learned people from this collective will have already come with simpler more accessible explanations.
Thanks People
Peace

 

[witwald]

Group delay is when the delay varies across frequency, so that the entire “group”, if you will, is arriving at different times relative to each other.

Group delay can also be constant as a function of frequency, and then it simply corresponds to a pure time delay

It is the derivative of phase (or maybe the integral).

Group delay is the negative derivative of the phase response.

 

[witwald]

In a box speaker, I always equated it with Booooooooooom!

Maybe that's totally wrong, but to me that's an easily audible artefact often exhibited in UK-porty domestic speakers...

To a large degree, I don't think that group delay can really be associated with the "boominess" of a loudspeaker system. Of course, a vented-box enclosure has a transient response that takes a bit longer to die down than that of a closed-box loudspeaker system, for the same cut-off frequency. As vented-box systems generally have more bass response than closed-box systems, that may be perceived by a listener as more "boom" when listened to in a room. However, there are some vented-box low frequency alignments that trade off greater low-frequency extension for a transient response that takes longer to settle. Those vented-box alignments should probably be avoided.

 

[witwald]

Minimum phase components arranged in series produce a minimum phase system.

Consider a loudspeaker driver, such as a tweeter. It is has a natural bandpass frequency response function, which is rolled off at low frequencies and at high frequencies. It turns out that if we measure the amplitude response of this tweeter, then it is possible to mathematically compute the phase response of the driver.

The required magnitude-to-phase transform only applies to so-called "minimum-phase systems". They are called minimum phase because the phase response is the minimum amount of phase shift that can be possessed by a system with that magnitude response.

If we now add a passive high-pass filter to the tweeter, the new filtered magnitude response of the tweeter is also minimum phase. Once again, the phase response can be obtained computationally from the magnitude response.

 

[audio2design]

Does this help?

Technical Support: N5242B PNA-X Microwave Network Analyzer

The PNA-X vector network analyzer offers the world's most integrated and flexible microwave test platform for active device characterization.

na.support.keysight.com

Whether or not it is a time delay, it is usually expressed mathematically as the derivative of phase with respect to frequency.

(Change of phase) / frequency = what? .... Time.

Some app engineer at Keysight copied something written somewhere else. That does not make it gospel wrt general usage.

Group delay is quite literally time delay. It seems a lot of people write group delay =

-d(phase)/d(frequency in radians)

without understanding they just wrote the formula for time delay at a given frequency. However as you saw in the Keysight article it talks about group delay being the average which takes me back to what I wrote ... It's the time delay of the amplitude response (on average).

 

[xaviescacs]

on average

Why on average? Can you explain that please?

 

[Raindog123]

Group delay is quite literally time delay

The question is what is it delay between?

-d(phase)/d(frequency in radians)

without understanding they just wrote the formula for time delay at a given frequency

You keep on saying “at a given frequency” - which is incorrect. At a given frequency the “d(frequency in radians)“ would be zero. A group delay is a [time or phase] delay _between_ various frequencies — either traveled through media or processed by, eg, a filter (while yes, it can be a function of frequency too).

In practical terms, it is the [propagation or processing] difference (the delay, the spread) between lower and higher [audio] frequencies.

I gave the group delay definition/meaning above, and it is correct.

 

[Jimbob54]

As long as they're all showing up at the same time, should not make a difference, n'est pas?

Clearly not been the anxious host of many parties then ;-)

 

[audio2design]

Why on average? Can you explain that please?

Because normally when people talk about group delay these either talk about it in rough terms, i.e. constant group delay, or they talk about it as a single number, i.e. an average. "Group" implies it applies to a group of something hence a single number would be the average for the group.

 

[pkane]

(Change of phase) / frequency = what? .... Time.

Some app engineer at Keysight copied something written somewhere else. That does not make it gospel wrt general usage.

Group delay is quite literally time delay. It seems a lot of people write group delay =

-d(phase)/d(frequency in radians)

without understanding they just wrote the formula for time delay at a given frequency. However as you saw in the Keysight article it talks about group delay being the average which takes me back to what I wrote ... It's the time delay of the amplitude response (on average).

d(phi)/d(f) is d(t) is the change in time.

Group delay usually refers to a constant/linear d(phi)/d(f) -- in other words, phase is a straight line when plotted on a linear frequency axis. This results in a simple (and intuitively understood) sound delay -- other than arriving a bit late, the sound is otherwise unchanged. If you record the sound starting at the exact time of its arrival, it will be exactly the same as the original sound.

More complex phase relationships, sometimes called variable group delay, are fairly common in digital filters and other DSP, cause the time delay to vary by frequency. d(phi)/d(f) is no longer a linear function. It can be an arbitrarily complex, non-linear expression.

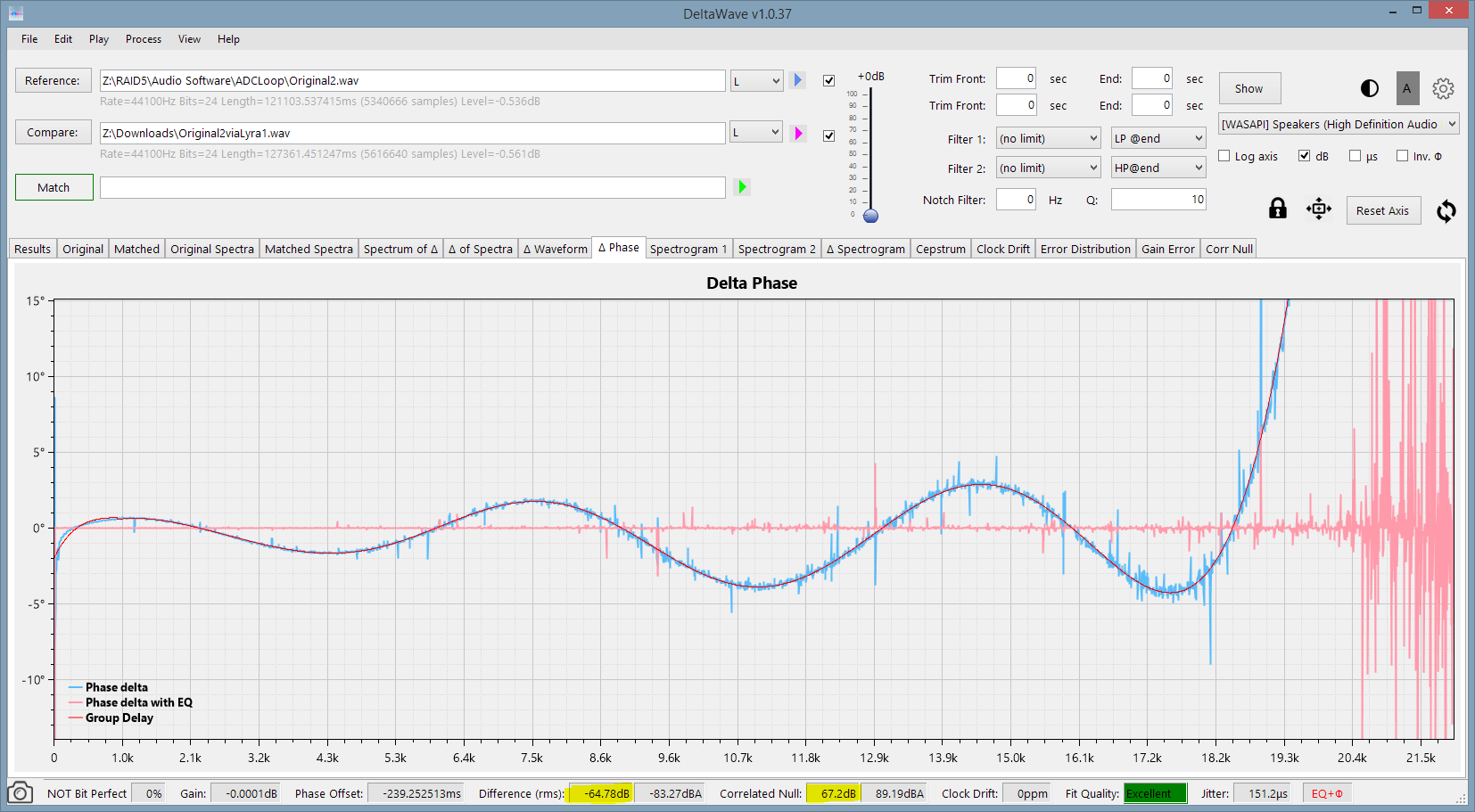
Here's what a non-linear phase (variable group delay) for one DAC looks like (blue). Red/pink/salmon line is what it looks like after phase is linearized. Since the slope of the red line is 0 (it's horizontal) there is no time delay. The sound recorded from such a variable group delay will not be exactly the same as the original sound, the waveform will be different. That's because different frequencies will be delayed by differing amounts of time.

 

[xaviescacs]

Because normally when people talk about group delay these either talk about it in rough terms, i.e. constant group delay, or they talk about it as a single number, i.e. an average. "Group" implies it applies to a group of something hence a single number would be the average for the group.

I more or less get what you mean intuitively, but I struggle to see this "average" in the mathematical definition. Thanks anyway.

 

[audio2design]

The question is what is it delay between?

You keep on saying “at a given frequency” - which is incorrect. At a given frequency the “d(frequency in radians)“ would be zero. A group delay is a delay _between_ various frequencies — either traveled through media or processed by, eg, a filter (while yes, it can be a function of frequency too).

The delay is between input and output however defined. Group delay is an absolute. It is not the delay between frequencies, it is the delay period. Written in derivative form, i.e. (d/dx)f(x) with x as frequency and f(x) as phase change at frequency.

What I wrote was derivative form. Group delay is a definition. It is the derivative of the phase response which as a function has an inherent frequency component and hence the calculation is at a frequency.

Group delay is a definition. Your definition was wrong whether you think the correct one sounds right or not.