Jean.Francois
Active Member
- Joined
- May 31, 2022
- Messages
- 152
- Likes
- 552
Hello,
“On Every Street” is the sixth and final studio album by the band Dire Straits, released on September 9, 1991. This album was released six years after the previous album, “Brothers in Arms”. “On Every Street” contains 12 tracks and was produced digitally.

For this review, 8 versions were tested: Vinyl record Back To Black and MOFI; CD from 1991,1996,2000 and MOFI; SACD MOFI and Tidal HD.
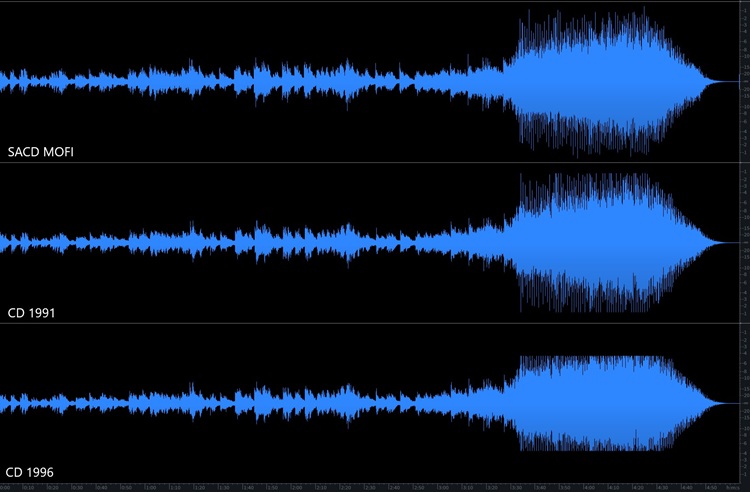
In terms of dynamics, it's the Mofi version that has the best dynamics compared to the 1991, 1996, 2000 and Tidal CD versions, as shown by the waveform zoom on the song "On Every Street" and the digital curves.
As far as the vinyl versions are concerned, one might wonder about the quality of vinyl realization today.
The graph below compares the spectra of Back To Black and MOFI vinyl records.
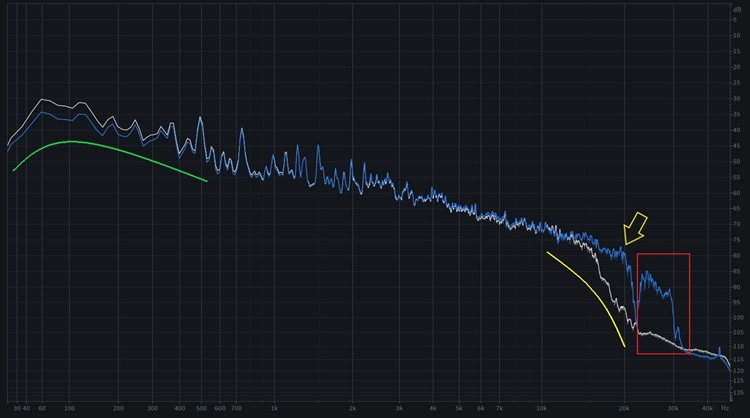
For the Back To Black vinyl record, the cutting was done by Bernie Grundman, and there is attenuation above 16 kHz (yellow zone), even though the music still contains information. We've been noticing a problem with Bernie Grundman's cutting for some time now, as was the case with the latest "Dark Side of The Moon". It's a real shame for today's productions, because in the past, the engravings were really of high quality, without these defects above 15 kHz.
On the MOFI vinyl record, the bandwidth rises well above 20 kHz (yellow arrow), with a present signal up to at least 30 kHz. However, in the red zone we see a signal that shouldn't exist, as the digital master cuts at 22 kHz. There's an aliasing of the spectrum, a phenomenon that shouldn't appear on a vinyl record and isn't present on other albums like "Brother In Arms", which also has a digital master. It's a digital flaw found on analog vinyl! You can find full details of this problem here.
To have a vinyl record with a high technical quality is not so simple as we can see.
To see the impact on vinyl records and compare all versions, you can listen to sample from the 8 versions HERE, and also find the measurements for all versions.
Enjoy listening,
Jean-François
“On Every Street” is the sixth and final studio album by the band Dire Straits, released on September 9, 1991. This album was released six years after the previous album, “Brothers in Arms”. “On Every Street” contains 12 tracks and was produced digitally.
For this review, 8 versions were tested: Vinyl record Back To Black and MOFI; CD from 1991,1996,2000 and MOFI; SACD MOFI and Tidal HD.
In terms of dynamics, it's the Mofi version that has the best dynamics compared to the 1991, 1996, 2000 and Tidal CD versions, as shown by the waveform zoom on the song "On Every Street" and the digital curves.
Waveform: CD 1991, CD 1996 and SACD MOFI
As far as the vinyl versions are concerned, one might wonder about the quality of vinyl realization today.
The graph below compares the spectra of Back To Black and MOFI vinyl records.
Spectrum Vinyl Back To Black - 2024 (white) vs Vinyl record Mofi - 2024 (blue)
For the Back To Black vinyl record, the cutting was done by Bernie Grundman, and there is attenuation above 16 kHz (yellow zone), even though the music still contains information. We've been noticing a problem with Bernie Grundman's cutting for some time now, as was the case with the latest "Dark Side of The Moon". It's a real shame for today's productions, because in the past, the engravings were really of high quality, without these defects above 15 kHz.
On the MOFI vinyl record, the bandwidth rises well above 20 kHz (yellow arrow), with a present signal up to at least 30 kHz. However, in the red zone we see a signal that shouldn't exist, as the digital master cuts at 22 kHz. There's an aliasing of the spectrum, a phenomenon that shouldn't appear on a vinyl record and isn't present on other albums like "Brother In Arms", which also has a digital master. It's a digital flaw found on analog vinyl! You can find full details of this problem here.
To have a vinyl record with a high technical quality is not so simple as we can see.
To see the impact on vinyl records and compare all versions, you can listen to sample from the 8 versions HERE, and also find the measurements for all versions.
Enjoy listening,
Jean-François