- Joined
- May 1, 2021
- Messages
- 427
- Likes
- 1,309
-Introduction-
May 20 I will give a presentation on the COMSOL Acoustics Day on state-of-the-art loudspeaker simulation techniques, and I will give ASR readers a little sneak peek here. As always, feel free to ask away in the thread, and I will try and answer as best I can.
-Loudspeaker Simulations-
There are many different simulations that are relevant for exploring loudspeaker behavior. Below I have made a rough overview of what typically is being requested.

So we can deal with single-physics (acoustics, structural mechanics, electromagnetics) or multi-physics problems (any combination of the beforementioned). And the study types can vary from static, to steady-state where you basically run a frequency sweep, to a modal analysis where you find eigenfunctions and eigenvalues of your system (see e.g. my post on Room Gain), to transient analyses where you apply a time-varying input and look at the transient and the steady-state response together.
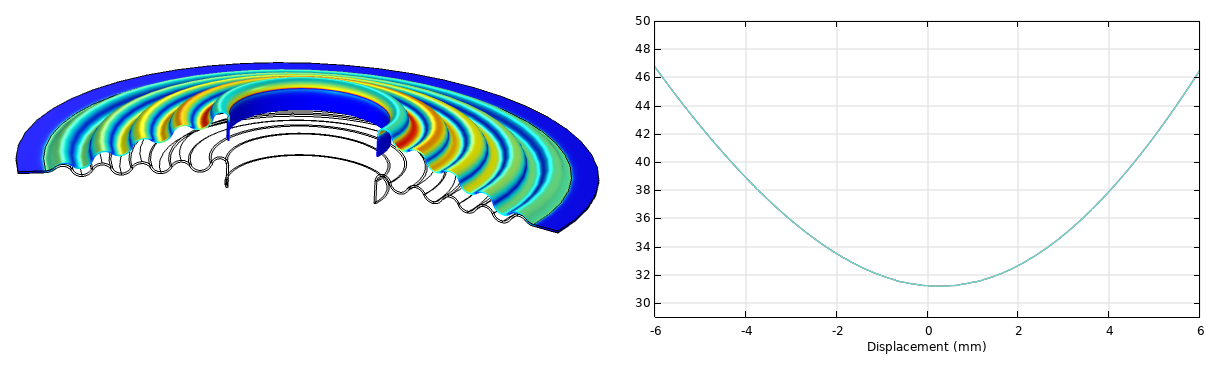
A static anaylysis could be relevant in conjunction with a structural mechanics to investigate the stiffness symmetry of spiders and surrounds as a function of displacement as shown below. The analysis takes into account the non-linear geometry, so that for small displacements the rolls 'unfold' with little strain, but at larger displacements you get more relative strain.
A typical steady-state problem would be a vibroacoustics (multi-physics) problem, where you model a complete driver and apply a harmonic voltage signal over a frequency range:
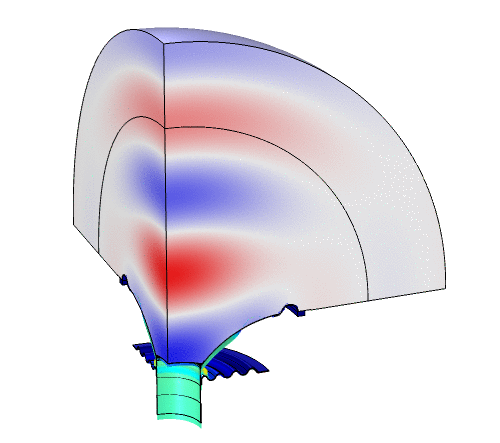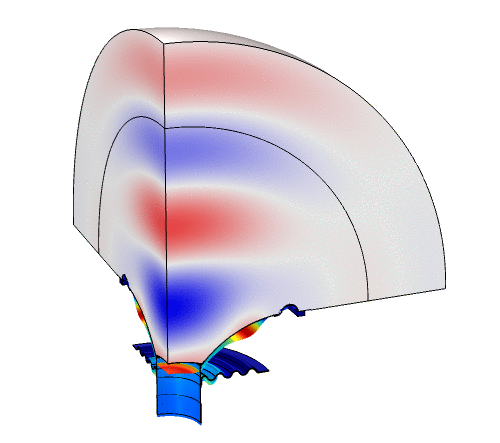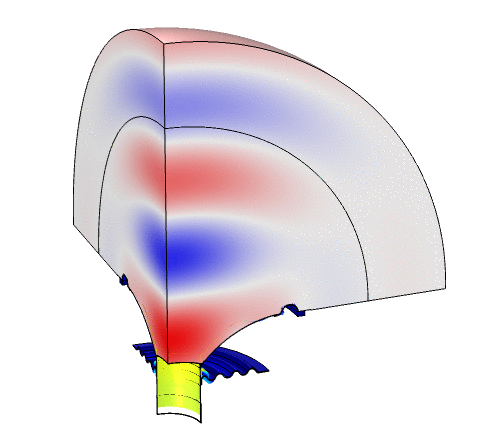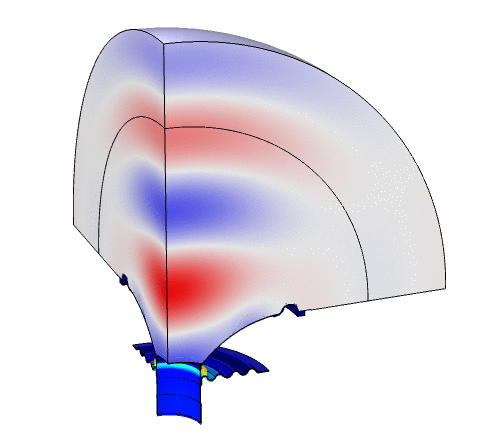
Assuming that all materical properties are correct, and the simulation in general is done correctly, you will get all information equivalent to Spinorama, plus much more. In the acoustics domain the only degree of freedom solved for is (complex valued) pressure, and that is all you need to calculate velocity, intensity, power, directivity index, and whatever else your heart pleases.
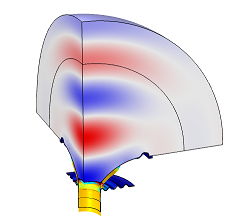
A modal analysis is relevant in several cases, for example to investigate the structural modes of a driver. Below a spider resonance is observed at a certain frequency, and so you can find all modes in the relevant frequency range and compare your findings to a steady-state frequency sweep to see if some modes lead to resonances.
Remember, modes exist independently of excitation, and not all modes are necessarily excited. So if you for example see a rocking mode in your modal analysis, you should remember how the excitation will work against such a mode.
Finally, transient responses can be relevant both to see the initial wave propagation, but also for investigating non-linear distortion coming primarily from the structural mechanics and electromagnetics. For the acoustics it can be relevant if you have high particle velocity/pressure, if you have small enclosures and drivers with large excursions, if you are looking at Doppler effects, and so on.
One technique that I have build into the software package COMSOL Multiphysics is Phase Decomposition, which allows me to dissect the cone and surround vibration to see which parts of the displacements add to, subtract from, or neither, the sound pressure in any observation point. A very underutilized technique in the loudspeaker industry.

Now, while the above analyses can be challenging enough in themselves, you can take things even further if start combining these analyses with formal, mathematical optimization. There are generally three groups of optimization, Parameter Optimization, Shape Optimization, and Topology Optimization (generally this is the ascending order of complexity).
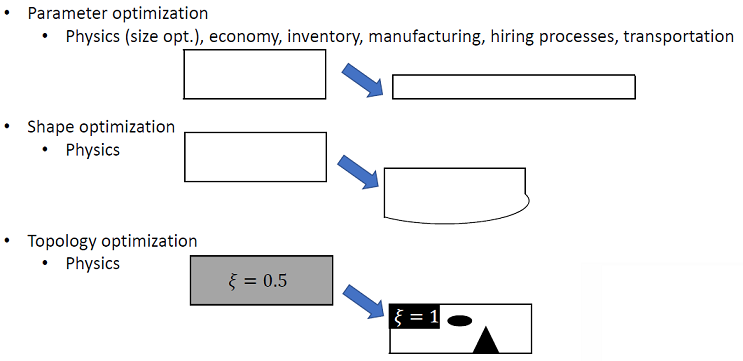
With Parameter optimization you can control the geometry via parameters such as lengths and heights, but basic shapes are retained. With Shape optimization boundaries are described in a way that allows the basic outline/shape to be controlled, but topology remains. Finally, with Topology optimization the topology of the geometry is allowed to change so that domains and holes are controlled completely by the algorithm. The underlying mathematics of multi-physics optimization is quite involved, so I will instead show the potential via some examples.
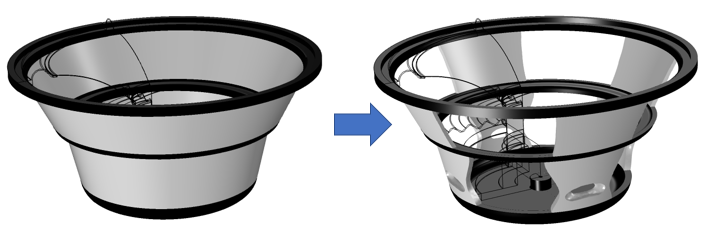
First is an acoustics shape optimization case where a compression driver geometry is optimized. This particular combination of physics and optimization can also be used for waveguide design, but the compression driver design has some more challenging aspects to it. The blue lines on the initial geometry are allowed to change shape, and the resulting geometry has the curved phase plug channels seen on the right, with a nice resulting pressure response.

Next, a generic setup with vibroacoustics and shape optimization. This was to explore how to do this particular multiphysics problem with shape optimization and it is rare to see any work with full vibroacoustics modelling with optimization included. I ended up using the Rayleigh integral for the acoustics, which made life a lot easier. The cone was allowed to change its shape, and the result was less relevant, as this was more of a test case.

I have also done shape optimization combined with the magnetics system, where a boundary was allowed to change its shape. Again, the results are less relevant for test cases than for actual client cases that I would probably not be allowed to show anyway.

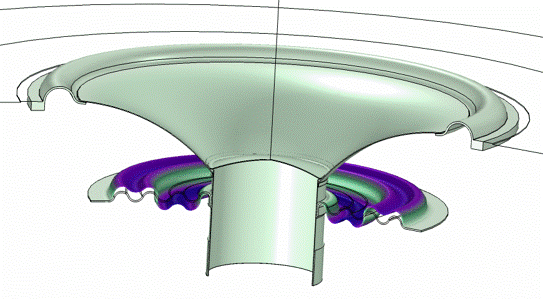
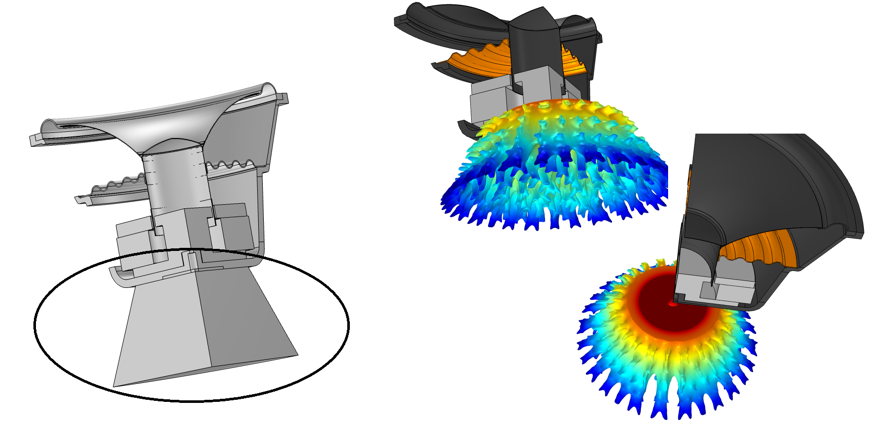
Moving into Topology optimization, here is a structural mechanics case, where I optimize the stiffness of a basket (for some given constraints). The holes that appear compared to the initial geometry completely grow out of the mathematics, which I find highly fascinating.
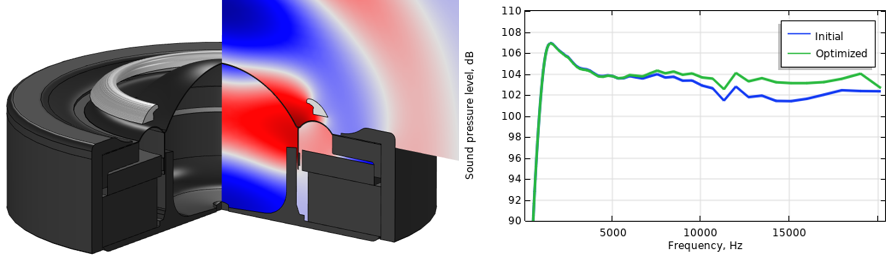
Next, an acoustic topology optimization where the complete multiphysics for the driver is included, but the optimization only takes place in the acoustics domain. With an objective to flatten the frequency response, a phase plug (in grey) has appeared in a domain in from of the driver assigned to be topology optimized. Again, this is a case I have never seen done before; including optimization in a full model of a realistic driver. It should of course be investigated how the off-axis response is affected, but imagine the time savings that are possible, compared to the traditional methods with clay modelling, and general trial and error with no guidance.
A final example is actual a heat conduction topology optimization case. As heat affects the material parameters of the structural mechanics and magnetics domains (and also the acoustics to some degree), it is desireable to lead heat away from the driver. So I thought this could be an interesting challenge. While I had never done any heat conduction cases, I spend a day reading relevant papers and setting up the simulation, and set the computer to work over the night. And I got this pretty heat sink.
-Closing remarks-
The above techniques are not all being utilized in the loudspeaker industry yet, but with the interest I am experiencing from several of them it will just be a matter of time, before we see more designs that are aided by formal optimization. Also, there are more simulations that I have not touched upon in the above, but I am working on composites with anistropic layer, metamaterials, and additional optimization cases, that will benefit the loudspeaker industry, so stay tuned.
- About me -
René Christensen, Denmark, BSEE, MSc (Physics), PhD (Microacoustics), FEM and BEM simulations specialist in/for loudspeaker, hearing aid, and consultancy companies. Own company Acculution, blog at acculution.com/blog
May 20 I will give a presentation on the COMSOL Acoustics Day on state-of-the-art loudspeaker simulation techniques, and I will give ASR readers a little sneak peek here. As always, feel free to ask away in the thread, and I will try and answer as best I can.
-Loudspeaker Simulations-
There are many different simulations that are relevant for exploring loudspeaker behavior. Below I have made a rough overview of what typically is being requested.
So we can deal with single-physics (acoustics, structural mechanics, electromagnetics) or multi-physics problems (any combination of the beforementioned). And the study types can vary from static, to steady-state where you basically run a frequency sweep, to a modal analysis where you find eigenfunctions and eigenvalues of your system (see e.g. my post on Room Gain), to transient analyses where you apply a time-varying input and look at the transient and the steady-state response together.
A static anaylysis could be relevant in conjunction with a structural mechanics to investigate the stiffness symmetry of spiders and surrounds as a function of displacement as shown below. The analysis takes into account the non-linear geometry, so that for small displacements the rolls 'unfold' with little strain, but at larger displacements you get more relative strain.
A typical steady-state problem would be a vibroacoustics (multi-physics) problem, where you model a complete driver and apply a harmonic voltage signal over a frequency range:
Assuming that all materical properties are correct, and the simulation in general is done correctly, you will get all information equivalent to Spinorama, plus much more. In the acoustics domain the only degree of freedom solved for is (complex valued) pressure, and that is all you need to calculate velocity, intensity, power, directivity index, and whatever else your heart pleases.
A modal analysis is relevant in several cases, for example to investigate the structural modes of a driver. Below a spider resonance is observed at a certain frequency, and so you can find all modes in the relevant frequency range and compare your findings to a steady-state frequency sweep to see if some modes lead to resonances.
Remember, modes exist independently of excitation, and not all modes are necessarily excited. So if you for example see a rocking mode in your modal analysis, you should remember how the excitation will work against such a mode.
Finally, transient responses can be relevant both to see the initial wave propagation, but also for investigating non-linear distortion coming primarily from the structural mechanics and electromagnetics. For the acoustics it can be relevant if you have high particle velocity/pressure, if you have small enclosures and drivers with large excursions, if you are looking at Doppler effects, and so on.
One technique that I have build into the software package COMSOL Multiphysics is Phase Decomposition, which allows me to dissect the cone and surround vibration to see which parts of the displacements add to, subtract from, or neither, the sound pressure in any observation point. A very underutilized technique in the loudspeaker industry.
Now, while the above analyses can be challenging enough in themselves, you can take things even further if start combining these analyses with formal, mathematical optimization. There are generally three groups of optimization, Parameter Optimization, Shape Optimization, and Topology Optimization (generally this is the ascending order of complexity).
With Parameter optimization you can control the geometry via parameters such as lengths and heights, but basic shapes are retained. With Shape optimization boundaries are described in a way that allows the basic outline/shape to be controlled, but topology remains. Finally, with Topology optimization the topology of the geometry is allowed to change so that domains and holes are controlled completely by the algorithm. The underlying mathematics of multi-physics optimization is quite involved, so I will instead show the potential via some examples.
First is an acoustics shape optimization case where a compression driver geometry is optimized. This particular combination of physics and optimization can also be used for waveguide design, but the compression driver design has some more challenging aspects to it. The blue lines on the initial geometry are allowed to change shape, and the resulting geometry has the curved phase plug channels seen on the right, with a nice resulting pressure response.
Next, a generic setup with vibroacoustics and shape optimization. This was to explore how to do this particular multiphysics problem with shape optimization and it is rare to see any work with full vibroacoustics modelling with optimization included. I ended up using the Rayleigh integral for the acoustics, which made life a lot easier. The cone was allowed to change its shape, and the result was less relevant, as this was more of a test case.
I have also done shape optimization combined with the magnetics system, where a boundary was allowed to change its shape. Again, the results are less relevant for test cases than for actual client cases that I would probably not be allowed to show anyway.
Moving into Topology optimization, here is a structural mechanics case, where I optimize the stiffness of a basket (for some given constraints). The holes that appear compared to the initial geometry completely grow out of the mathematics, which I find highly fascinating.
Next, an acoustic topology optimization where the complete multiphysics for the driver is included, but the optimization only takes place in the acoustics domain. With an objective to flatten the frequency response, a phase plug (in grey) has appeared in a domain in from of the driver assigned to be topology optimized. Again, this is a case I have never seen done before; including optimization in a full model of a realistic driver. It should of course be investigated how the off-axis response is affected, but imagine the time savings that are possible, compared to the traditional methods with clay modelling, and general trial and error with no guidance.
A final example is actual a heat conduction topology optimization case. As heat affects the material parameters of the structural mechanics and magnetics domains (and also the acoustics to some degree), it is desireable to lead heat away from the driver. So I thought this could be an interesting challenge. While I had never done any heat conduction cases, I spend a day reading relevant papers and setting up the simulation, and set the computer to work over the night. And I got this pretty heat sink.
-Closing remarks-
The above techniques are not all being utilized in the loudspeaker industry yet, but with the interest I am experiencing from several of them it will just be a matter of time, before we see more designs that are aided by formal optimization. Also, there are more simulations that I have not touched upon in the above, but I am working on composites with anistropic layer, metamaterials, and additional optimization cases, that will benefit the loudspeaker industry, so stay tuned.
- About me -
René Christensen, Denmark, BSEE, MSc (Physics), PhD (Microacoustics), FEM and BEM simulations specialist in/for loudspeaker, hearing aid, and consultancy companies. Own company Acculution, blog at acculution.com/blog
Last edited:
